(a) Sexagesimal (or English) System:
 System.jpg)
(b) Centesimal (OR French System):
.jpg)
(c) Circular System:
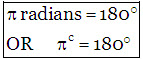
► Relation between three systems of measurement of angle
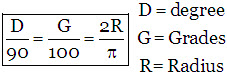
Area of a Sector of a Circle:
► Let AOB be a sector of a circle, centre O, radius r. Let ∠APB = θ radian arc AB = l. Then Area of the sector AOB = (1/2)r2 θ.

Trigonometric Function:
(1) Fundamental Identities
sin2 θ + cos2 θ = 1
1 + tan2 θ = sec2 θ
1 + cos2 θ = cosec2
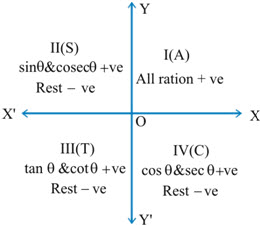
(2) Signs of Trigonometric ration is quadrants
► Angle θ & (90◦ – θ◦) are complementary angles, θ & (180◦ – θ◦) are supplementary angles.
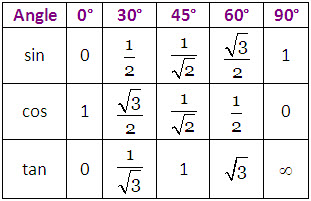
.jpg)
.jpg)
cos n π = (–1)n, n ∊ l
sin π = 0, n ∊ l
cos (nπ/2) = 0 if n is odd integer
cos(nπ + θ) = (–1)n cos θ, if n ∊ l
cos(nπ + θ) = (–1)n sin θ, if n ∊ l
cos(2nπ ± θ) = cos θ, n ∊ l
tan(nπ ± θ) = tan θ, n 0 ∊ l
Trigonometric Ratio of Compound Angles:
(1) sin(A ± B) = sin A cos B ± cos A sin B
cos(A ± B) = cos A cos ∓ sin A sin B
tan (A ± B) = (tan A ± tan B)/(1 ∓ tan A tan B)
(2) sin 2A = 2 sin A cos A = (2 tan A)/(1 + tan2 A)
cos 2A = cos2 A – sin2 A = 2 cos2 A – 1 = 1 – 2 sin2 A = ((1 – tan2 A) / (1 + tan2 A)) tan 2A = (2 tan A)/(1 – tan2 A)
(3) ![]()
![]()
![]()
![]()
Sum and Difference Formulae:
(1) ![]()
(2) ![]()
![]()
(3) sin(A + B) sin (A – B) = sin2 A – sin2 B = cos2 B – cos2 A
(4) cos(A + B) cos(A – B) = cos2 A – sin2 B
(5) sin 3θ = 3 sin θ – 4 sin3 θ
(6) cos 3θ = 4 cos3 θ – 3 cos θ
(7) ![]()
(8) ![]()
(9) ![]()
(10) ![]()
Product into Sum or Difference:
► 2 sin A cos B = sin (A + B) + sin (A – B)
► 2 cos A sin B = sin (A + B) – sin (A – B)
► 2 cos A cos B = cos (A + B) + cos (A – B)
► 2 sin A sin B = cos (A – B) – cos (A + B)
T-Ratios of the Sum of Three or more Angles:
► sin (A + B + C) = sin A cos B cos C + cos A sin B cos C + cos A cos B sin C – sin A sin B sin C
OR
sin (A + B + C) = cos A cos B cos C (tan A + tan B + tan C – tan A tan B tan C)
► cos (A + B + C) = cos A cos B cos C – sin A sin B cos C – sin A cos B sin C – cos A sin B sin C
OR
cos (A + B + C) = cos A cos B cos C (1 – tan A tan B – tan B tan C – tan C tan A)
► ![]()
► If A + B + C = π, then
(a) sin 2A + sin 2B + sin 2C = 4 sin A sin B sin C
(b) sin A + sin B + sin C = 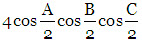
(c) cos 2A + cos 2B + cos 2C = –1 – 4 cos A cos B cos C
(d) cos A + cos B + cos C = .jpg)
(e) .jpg)
(f) tan A + tan B + tan C = tan A tan B tan C
(g) .jpg)
(h) cot A cot B + cot B cot C + cot C cot A = 1
(i) tan A tan B + tan B tan C + tan C tan A = 1 + sec A sec B sec C
► The solution consisting of all possible solutions of a trigonometric equation is called its general solution.
sin θ = 0 ⇒ θ = nπ
cos θ = 0 ⇒ θ = (2n + 1)(π/2)
tan θ = 0 ⇒ θ = nπ
sin θ = sin α ⇒ θ = nπ + (–1)n, α, α ∊ [(–(π/2)), (π/2)]
cos θ = cos α ⇒ θ = 2nπ ± α; α ∊ [0, π]
tan θ = tan α ⇒ θ = nπ + α; α ∊ [(–(π/2)), (π/2)]
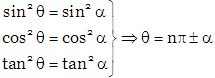
Principal Value:
Numerically least value of the angle is called its principal value.